Have you heard about the shape, Quadrilateral? No!. Let me tell you, a quadrilateral is a type of geometrical shape which is bounded by four sides. There are various types of quadrilateral such as square, rectangle, rhombus, and so on. One of the subtypes of a quadrilateral is the trapezium. It can be defined as the convex quadrilateral which has one pair of parallel and one pair of non-parallel sides respectively. A trapezium can be considered as a two-dimensional shape that has both dimensions, length, and breadth. When we draw a trapezium on a table, it may appear as a table with four legs. The trapezium has four sides, vertices, and angles respectively. Thus, it can also be regarded as a polygon. Generally, it is also known as a trapezoid. In this article, we shall cover some basic topics regarding trapezium such as properties of trapezium, area, and perimeter, and do a detailed discussion on these topics.
Area of Trapezium
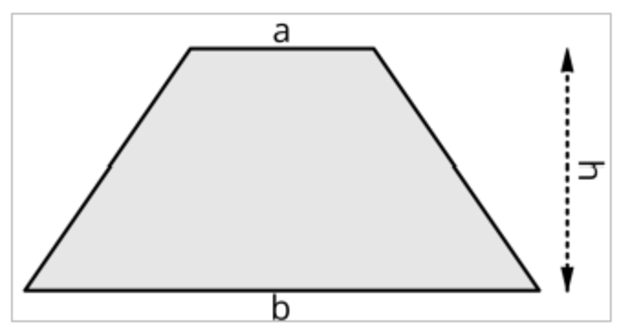
The maximum number of square units a trapezium can fill inside it can be defined as the area of trapezium or trapezoid. The square units signify, meter square, centimeter square, in. in (in = inches). The parallel sides of the trapezium are also known as the bases of the trapezium and the non-parallel sides as the legs of the trapezium. The area of trapezium can be calculated using various formulas. Such as, it can use a parallelogram to calculate its area, we can divide a trapezium into two triangles and find its area. But, the most common and basic formula is, ½ * a + b * h where a and b can be denoted as the bases of trapezium and h is the height of the trapezium. The resultant answer or value is always written in square units. It is not possible to draw the square units of the shape and find the area of trapezium. Hence, you can use the formulas mentioned above. We shall solve some examples based on them in the coming sections. Types of trapezium
Properties of Trapezium
As mentioned above, a trapezium can be considered as a two-dimensional shape that has both dimensions, length, and breadth. The following are the properties of a trapezium in a detailed manner.
- A trapezium is made up of 4 sides, angles, and vertices respectively. Vertices are the plural form of the vertex. It can be defined as the highest point of a trapezium.
- A trapezium consists of one pair of parallel and non-parallel sides respectively. The parallel sides of the trapezium are also known as the bases of the trapezium and the non-parallel sides as the legs of the trapezium.
- Diagonals of a trapezium always intersect each other.
- A trapezium is classified into three types: isosceles trapezium, scalene trapezium and right trapezium. They are classified based on the parallel and non-parallel sides. For example, if the measurement of the non-parallel sides is equal, they are considered as isosceles trapezium.
- The midpoint of the trapezium is always parallel to the bases of a trapezium. It is written as, AB + CD/2 where AB and CD are regarded as the bases or parallel sides of a trapezium.
- The mathematical formula to find the area of trapezium is ½ * a + b * h where a and b are the parallel sides and h is the height of trapezium.
- The mathematical formula given for the perimeter of the trapezium is, a + b + c + d where the alphabets i.e a, b, c, and d signify the sides of the trapezium. Click here:
Example 1: Find the Area of the Trapezium if the length of the sides is 8 and 6 cm and height is 9 cm.
Given that,
Bases of the trapezium = 8 and 6 cm ( a = 8 and b = 6 ).
Height of the trapezium = 9 cm.
Using the formula = ½ * a + b * h
½ * ( 8 + 6 ) * 9 = ½ * 14 * 9
½ * 14 * 9 = 63 cm square units.
Hence, the area of trapezium is = 63 cm square units.
Visit Cuemath to study more amazing concepts.